Calculating the reflectivity
For a set of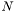 slabs, that run from the front layer that the neutrons enter, (
slabs, that run from the front layer that the neutrons enter, (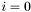 ), to the bulk layer at the back, (
), to the bulk layer at the back, (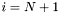 ), reflectivity can be calculated by the Parratt formalism. The measured wavevector transfer
), reflectivity can be calculated by the Parratt formalism. The measured wavevector transfer 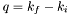 value is determined at scan time by the angle of the scan and the wavelength:
value is determined at scan time by the angle of the scan and the wavelength: 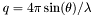 . The incident wavevector normal to the surface in a layer (
. The incident wavevector normal to the surface in a layer ( ) is related to that in vacuum (
) is related to that in vacuum (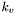 ) by
) by
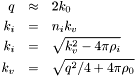
where 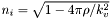 is the index of refraction for a layer with total scattering length density
is the index of refraction for a layer with total scattering length density 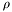 .
.
The Fresnel reflectiviy between any two layers is given by
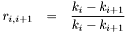
The total reflectivity is calculated from back to front assuming there is no refelctivity from the backmost (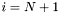 ) layer (
) layer (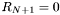 ) since it is infinite in extent.
) since it is infinite in extent.
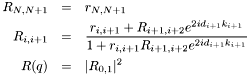
The reflectivity of the entire system is given by the reflectivity from the top surface, and is shown in the last line above. Here,  and
and 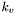 are real valued, and everything else, including
are real valued, and everything else, including 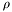 , is complex.
, is complex.
 1.5.5
1.5.5